Statistical consequences of using bone mineral density to pair cadaver femurs in comparative ex vivo hip fracture studies

Article focus

Using human cadaver femurs is regarded as the gold standard in ex vivo hip fracture fixation studies

When pairs of femurs from the same donor cannot be achieved, pairing according to bone mineral density (BMD) is sometimes performed

The aim of this study was to evaluate the statistical consequences of pairing cadaver femurs according to BMD in ex vivo hip fracture fixation experiments when compared with donor pairs

Key messages

Compared with donor pairs, the intra-pair variance in BMD-matched pairs increased considerably and the intra-pair correlation was not significant

The required sample size to achieve a statistical power of 0.8 increased from ten pairs using donor pairs to 54 pairs using BMD-matched pairs

Paired statistics are no longer valid when the femurs are matched according to BMD

Strengths and limitations

Strengths: the test setup was designed to recreate the clinical situation and not to test the extremes

This statistical experiment clearly shows the importance of creating comparable test groups in ex vivo biomechanical experiments that traditionally include a limited number of specimens

Limitation: the study only evaluates pairing according to BMD and donor and not other possible methods of pairing femurs

Introduction

Paired designs are chosen in comparative experiments in order to minimise the effects of femur variation and thereby create comparable test groups. The sample variance is crucial in comparative statistics as increased variance within a given sample decreases statistical power. Consequently, restricting variability within pairs used in comparative studies reduces the required sample size, which is most welcomed amongst researchers working with limited resources such as cadaver bones.

The femoral size, neck–shaft angle, neck and head version and other geometrical factors show great variation between individuals.¹ In addition, the relative distribution of cortical and trabecular bone and bone porosity change with ageing.^2,3 All of these factors influence the biomechanical properties of human femurs and have the potential to affect the outcomes in experimental hip fracture studies. Using a paired study design, left and right femurs from the same donor can be randomly assigned to one of two modes of treatment.^4-8 If paired femurs from the same donor cannot be obtained, or when more than two groups are to be compared, pairing the femurs according to bone mineral density (BMD) seems to be the method of choice.^9-11

In a previous study we compared implant A (three screws in an inverted triangle configuration locked in a lateral support plate) and implant B (three screws in an inverted triangle configuration) when used for fixation of fractures of the femoral neck.¹² The femurs were paired by donors with the implants randomly allocated to the left or right femur. The main finding was that implant A slightly but significantly improved the stability of the construct. We present here a re-analysis of the obtained data following splitting of the donor pairs with subsequent new pairing according to BMD. The statistical effects were evaluated to see if this mode of matching could produce statistical strength equal to that of donor pairs.

Materials and Methods

For a detailed description of the experimental setup we refer to a previously published paper.¹² After receiving approval from the Regional Committee for Medical and Health Research Ethics, 18 fresh-frozen femurs from nine donors underwent Dual Energy X-Ray Absorptiometry (DXA) to obtain femoral neck BMD (Lunar iDXA, GE Healthcare, Madison, Wisconsin). Standardised subcapital fractures of the femoral neck were fixed using implant A or implant B and the femurs were tested in a hip simulator. The femurs completed 10 000 cycles of subject-specific axial load and torque based on the weight of the donor (joint resultant force 2.5 × bodyweight; sd 0.2). Three-dimensional migrations of the femoral head fragments were captured by an optical motion tracker system (Polaris Spectra, NDI, Ontario, Canada). Femoral head fragment migration described the stability of the fracture fixation. In group A, femoral pairs were matched according to donor. In group B, each femur was paired to the femur with the closest match in BMD other than its biological counterpart. For example, in donor pair 1, the two femurs had BMDs of 0.839 g/cm² and 0.928 g/cm², respectively. The femur allocated to implant group A was then matched to the femur in implant group B with a BMD of 0.887 g/cm², which was the closest match.

Results

Stability of the femoral head fragment

The mean paired difference in head fragment migration using implant A and implant B was 1.6 mm. The sd (mm) increased from 2.0 to 5.0 when paired according to BMD (p = 0.118).

The correlation of femoral head fragment migrations within pairs was highly significant when using donor pairs (Pearson’s r 0.953, p < 0.000). This correlation was lost in BMD-matched pairs (Pearson’s r -0.134, p = 0.730) (Fig. 1) (Fig. 1).

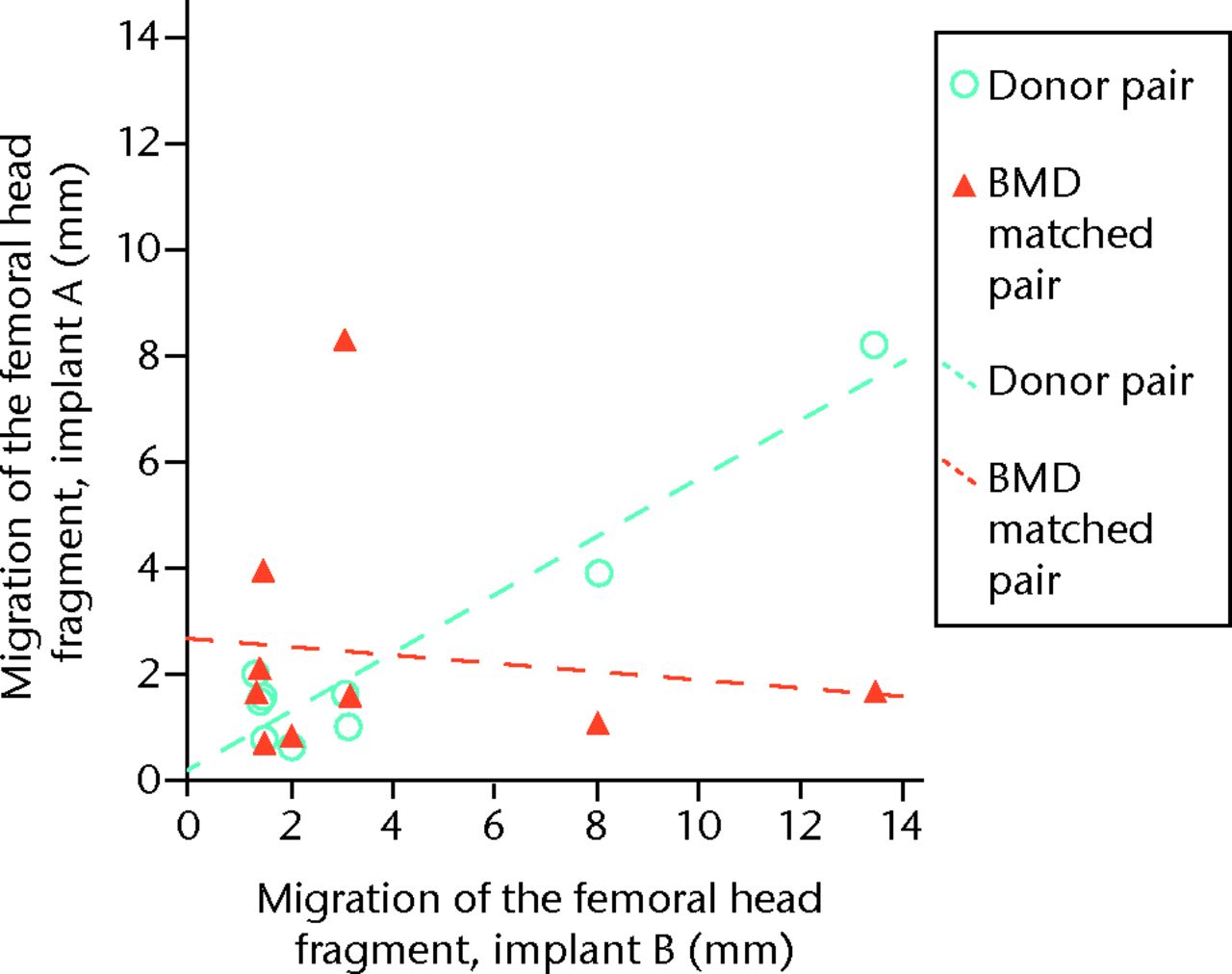
Fig. 1

Graph showing intra-pair correlations of femoral head fragment migrations.

Using donor pairs, the difference of 1.6 mm in femoral head fragment migration between implant group A and group B reached statistical significance (95% CI 0.1 to 3.1, p = 0.040). In BMD-matched pairs this difference was not significant (95 % CI -2.27 to 5.47, p= 0.368) (Fig. 2) (Fig. 2).

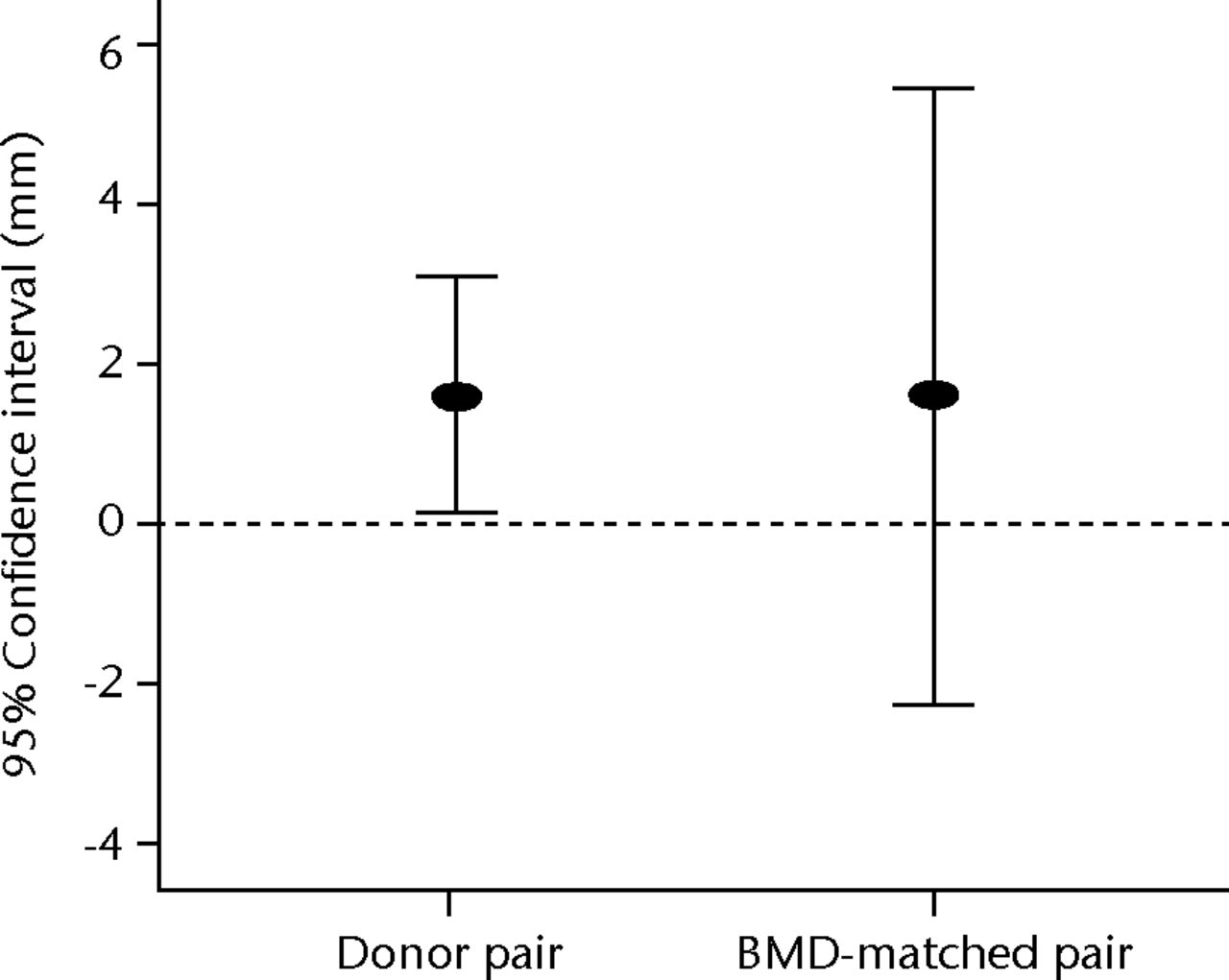
Fig. 2

Graph showing the slightly statistically significant difference between the two implant groups using donor pairs, which is lost in BMD-matched pairs due to increased variance.

The sds of the difference in femoral head fragment migration in donor pairs and in BMD-matched pairs were used to calculate the hypothetical sample size required to test a difference in migration of 2 mm. To obtain statistical power of 80%, ten pairs were needed when using donor pairs and 54 pairs were needed when using BMD-matched pairs (Fig. 3).

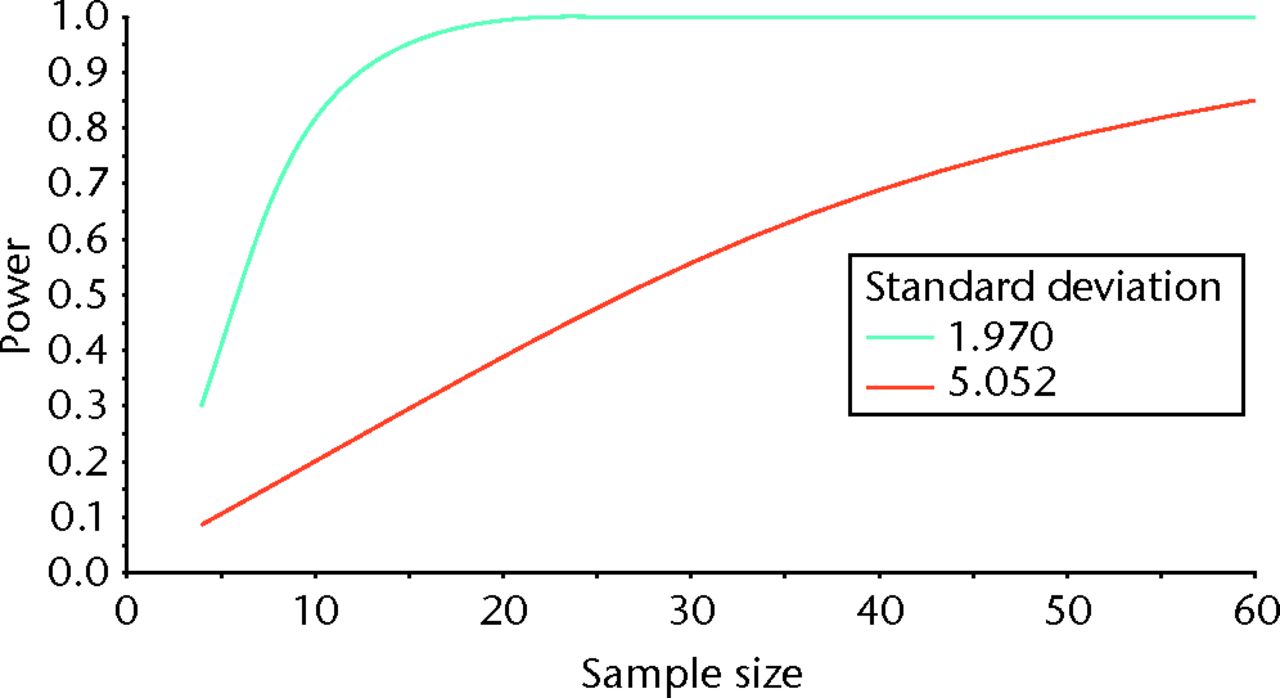
Fig. 3

Graph showing sample size estimation for BMD-matched pairs (orange line) and donor pairs (green line).

Discussion

Re-pairing donor pairs according to BMD did not change the within-group variance in BMD. Consequently, the observed differences within and between donor pairs and BMD-matched pairs cannot be explained by an initial difference in BMD.

The sample size in this experiment was small but reflects the number of femurs often used in biomechanical hip fracture experiments, regardless of the mode of matching. This is reflected by all of the comparative studies cited here. However, the results from this statistical experiment are rather unambiguous: after operating the fractures of the femoral neck with implant A or implant B and testing the constructs for fixation stability, the related sample correlation was 0.953 when using donor pairs. This correlation was -0.134 and non-significant in the BMD-matched pairs. Consequently, paired statistics can no longer be used and statistics for independent samples must be performed instead. When adding that the sd increased from 2 mm to 5 mm following re-pairing, the results showed that a fivefold increase in sample size was needed to compensate for this when seeking a statistical power of 80%. Ignoring this when setting up hip fracture experiments increases the risk of missing an existing effect (type II error).

The mechanical property of the human femur is not only determined by its microstructure such as bone mineral content. Femoral size, neck length, neck diameter, neck–shaft angle and anteversion angle must also be considered important factors in providing stable bone-implant constructs. Matching the pairs according to BMD alone does not take into account these factors as two anthropometrically different femurs might have similar BMDs.

In conclusion, pairing femurs according to BMD does not appear to be a good alternative to donor-matched pairs as the intra-pair variances increase. When more than two methods are to be compared, or if paired femurs from the same donor cannot be obtained, the sample size must be increased substantially to provide valid results.